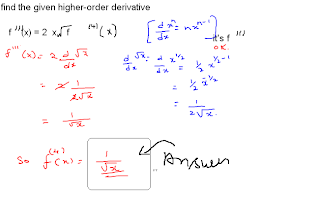
Saturday, March 24, 2012
Friday, March 23, 2012
Twelve sheep were fed a special diet consisting of dried grass and barley for three 2. months. At the end of the period the plasma insulin concentration (U/ml) was determined for each sheep. The data are as follows:20.5 26.2 18.8 21.2 22.1 21.6 25.4 26.8 22.9 22.8 30.8 28.4 What does the IQR explain about the distribution of the plasma insulin concentration of the twelve sheep?, c) Five number summary. Also construct the boxplot for the above data set.
"Twelve sheep were fed a special diet consisting of dried grass and barley for three 2. months. At the end of the period the plasma insulin concentration (U/ml) was determined for each sheep. The data are as follows:20.5 26.2 18.8 21.2 22.1 21.6 25.4 26.8 22.9 22.8 30.8 28.4 Calculate the following:a) First (Q1) and third (Q3) quartiles, b) The interquartile range (IQR). "
calculate the PH of a solution formed by mixing 85 ml of 0.13 M lactic acid with 95 ml of 0.15 M sodium lactate. (K HLaC =1.4 * 10^-4)
Ans:-
this is a buffer soluton of lactice acid and its conjugate base
moles
of salt = 95*0.15
= 14.25 mili
moles
reaction
of salt
NaC3H5O3 <---> Na+
+ C3H5O3 -
[C3H5O3-]= 14.25 mili moles
moles
of acid = 85*0.13
= 11.05 mili moles
Henderson Hasselbalch equation for a weak acid
pH = Pka + log
([A¯] / [HA])
so
formula change to
pH = Pka + log
([ C3H5O3¯]
/ [C3H6O3])
plug
the value in formula, we get
pH
= - log KHlac + log 14.25/11.05
pH
= - log 1.4 * 10^-4 + log 14.25/11.05
use
calculator to solve these values, we get
pH
= 3.85+ 0.11
pH
=3.96
Answer = pH of solution is 3.96
In this problem we have learned
graph each linear equation in two variable. Find at least five solutions in your table of values for each equation. y = 1/3x -1 and y=-5/2x +1
graph each linear equation in two variable. Find at least five solutions in your table of values for each equation. y = 1/3x -1 and y=-5/2x +1
Answer :-
In first equation y = 1/3x -1 the cofficient of x is 1/3
so plug the value multiply of 3 so plug x = 0,-3,+3,-6,+6 we get
| x | (x/3) -1 |
| 0 | -1 |
| -3 | -2 |
| 3 | 0 |
| -6 | -3 |
| 6 | 1 |
in second equation y=-5/2x + 1 the cofficient of x is -5/2 here 2 is in denominator
so plug the value x multiple of 2 so plug x = 0 ,-2 , 2, 4, -4
| x | (-5/2)*x +1 |
| 0 | 1 |
| 2 | -4 |
| -2 | 6 |
| 4 | -9 |
| -4 | 11 |
now use these values to draw the graph
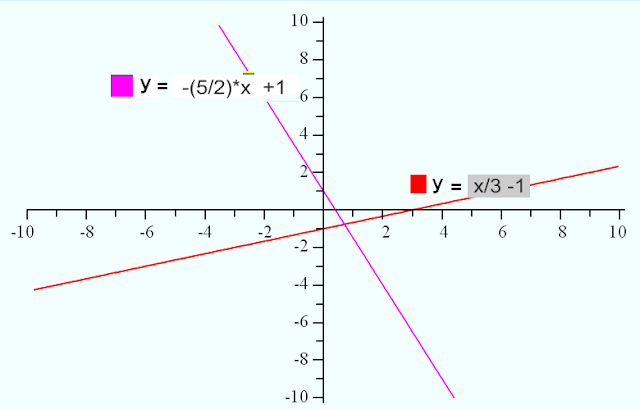 |
| graph each linear equation in two variable. Find at least five solutions in your table of values for each equation. y = 1/3x -1 and y= -5/2x +1 |
with help of this problem you can easily under stood
How to solve graph the linear equations ?
how to find the solution table for the equaions ?
how to draw the graph using equations?
derivative of sin(sin(sinx))
derivative of sin(sin(sinx)), example of chain rule, how to solve a chain rule problem?, solve the derivative using the chain rule
derivative of sin(sin(sin x))
use formula of chain rule
=>d/dx (sin y) = cos y * dy/dx
the value of y= sin (sin x)
use above formula we get
=> cos (sin (sinx))*d/dx (sin(sin x))
now new value of y = sinx
use above formula again we get
=> cos (sin (sin x))* cos(sin x) * d/dx sin x
now use the same formula again we get
=> cos (sin (sin x))* cos(sin x) * cos x
derivative of sin(sin(sin x))
use formula of chain rule
=>d/dx (sin y) = cos y * dy/dx
the value of y= sin (sin x)
use above formula we get
=> cos (sin (sinx))*d/dx (sin(sin x))
now new value of y = sinx
use above formula again we get
=> cos (sin (sin x))* cos(sin x) * d/dx sin x
now use the same formula again we get
=> cos (sin (sin x))* cos(sin x) * cos x
Answer = cos (sin (sin x))* cos(sin x) * cos x
Subscribe to:
Comments (Atom)